a) \(x^2+2x\sqrt{x-\dfrac{1}{x}}=3x+1\left(1\right)\)
Dễ dàng nhận thấy x=0 không phải là nghiệm của phương trình (1).
Chia cả hai vế của phương trình (1) cho x ta được:
\(x+2\sqrt{x-\dfrac{1}{x}}=3+\dfrac{1}{x}\)
\(\Leftrightarrow\left(x-\dfrac{1}{x}\right)+2\sqrt{x-\dfrac{1}{x}}-3=0\)
Đặt \(a=\sqrt{x-\dfrac{1}{x}}\left(a\ge0\right)\). Khi đó phương trình trở thành:
\(a^2+2a-3=0\Leftrightarrow\left[{}\begin{matrix}a=1\left(n\right)\\a=-3\left(l\right)\end{matrix}\right.\Rightarrow a=1\)
\(\Rightarrow\sqrt{x-\dfrac{1}{x}}=1\Leftrightarrow x-\dfrac{1}{x}=1\Leftrightarrow x^2-x-1=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1+\sqrt{5}}{2}\\x=\dfrac{1-\sqrt{5}}{2}\end{matrix}\right.\)
Thử lại ta có nghiệm của phương trình (1) là \(x=\dfrac{1+\sqrt{5}}{2}\) và \(x=\dfrac{1-\sqrt{5}}{2}\)
b) \(\left\{{}\begin{matrix}a,b,c>0\\a+b+c=1\end{matrix}\right.\)
Áp dụng bất đẳng thức Cô-si: \(ab\le\dfrac{\left(a+b\right)^2}{4}\)
\(\Rightarrow\dfrac{a+b}{abc}\ge\dfrac{4\left(a+b\right)}{\left(a+b\right)^2c}=\dfrac{4}{\left(a+b\right)c}\)
Lại áp dụng bất đẳng thức Cô-si: \( \left(a+b\right)c\le\dfrac{\left[\left(a+b\right)+c\right]^2}{4}=\dfrac{1}{4}\)
\(\Rightarrow\dfrac{4}{\left(a+b\right)c}\ge\dfrac{4}{\dfrac{1}{4}}=16\) \(\Rightarrow P=\dfrac{a+b}{abc}\ge16\)
Dấu "=" xảy ra khi \(\left\{{}\begin{matrix}a=b;a+b=c\\a+b+c=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=b=\dfrac{1}{4}\\c=\dfrac{1}{2}\end{matrix}\right.\)
Vậy \(MinP=16\)






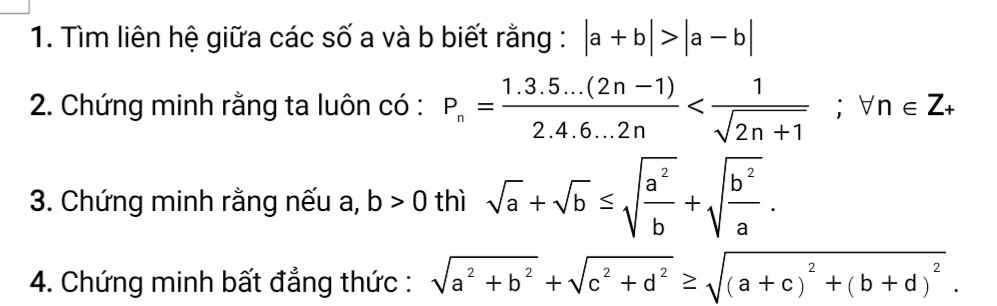


 Mọi người giúp mình với, giải chi tiết (+giải thích giúp mình nha), cảm ơn mọi người rất nhiều lun =)
Mọi người giúp mình với, giải chi tiết (+giải thích giúp mình nha), cảm ơn mọi người rất nhiều lun =)
