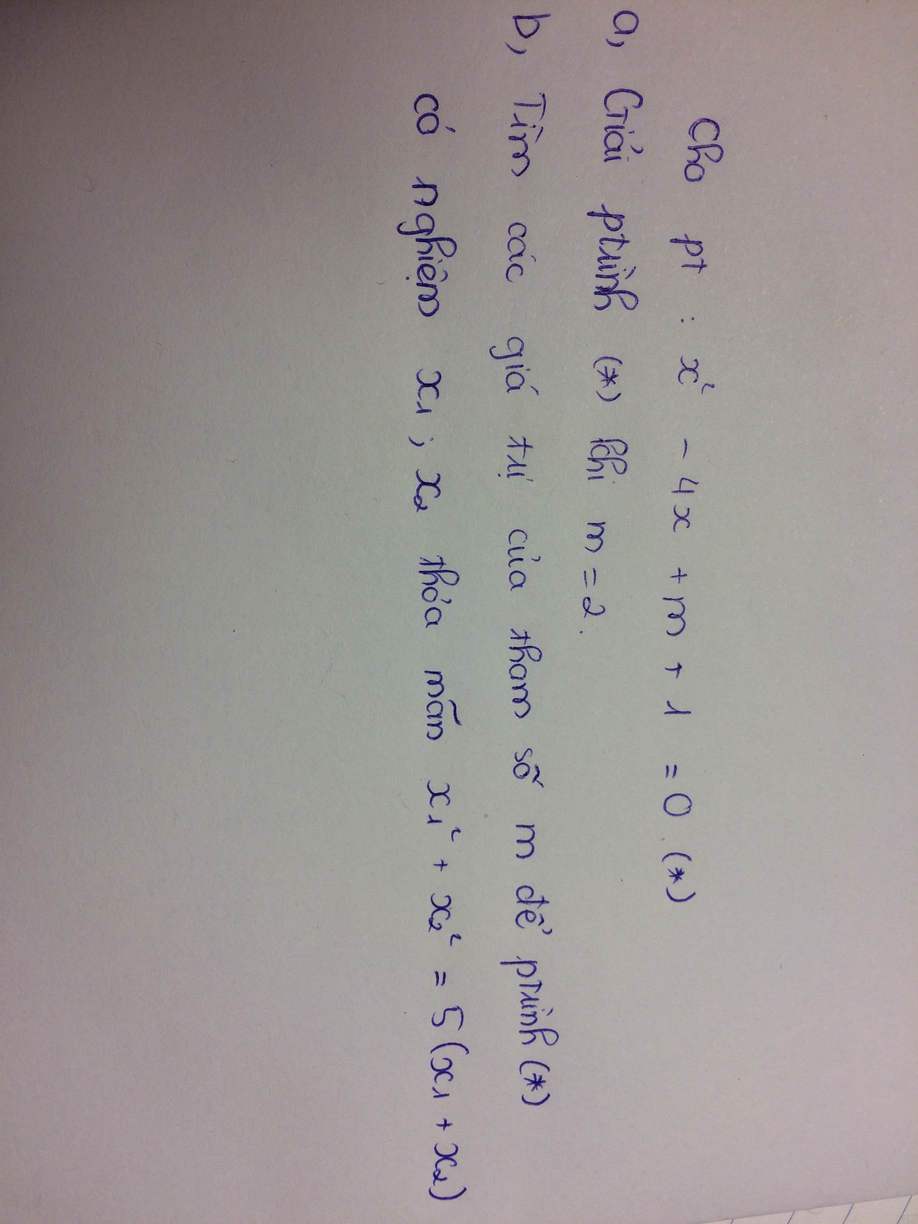\(\Delta'=4-\left(m+1\right)\ge0\Rightarrow m\le3\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=4\\x_1x_2=m+1\end{matrix}\right.\)
\(x_1^2+x_2^2=5\left(x_1+x_2\right)\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=5\left(x_1+x_2\right)\)
\(\Leftrightarrow16-2\left(m+1\right)=20\)
\(\Leftrightarrow m=-3\) (thỏa mãn)
a. Ta có: \(x^2-4x+m+1=0\)
Thay m=2 ta được: \(x^2-4x+2+1=0\Leftrightarrow x^2-4x+3=0\Leftrightarrow\left[{}\begin{matrix}x=3\\x=1\end{matrix}\right.\)
b. Để phương trình có 2 nghiệm phân biệt thì \(\Delta=\left(-4\right)^2-4.1.\left(m+1\right)>0\)
\(\Leftrightarrow16-4\left(m+1\right)>0\Leftrightarrow16>4\left(m+1\right)\Leftrightarrow4>m+1\Leftrightarrow m< 3\)
Áp dụng định lí Vi-et ta có: \(\left\{{}\begin{matrix}x_1+x_2=4\\x_1x_2=m+1\end{matrix}\right.\)
Theo đề ta có: \(x_1^2+x_2^2=5\left(x_1+x_2\right)\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=5\left(x_1+x_2\right)\)
\(\Leftrightarrow\left(4\right)^2-2\left(m+1\right)=5.4\)
\(\Leftrightarrow16-2m-2=20\Leftrightarrow m=-3\) (TM)
E lm đc phần a r ạ còn phần b nó cứ lú lú ý