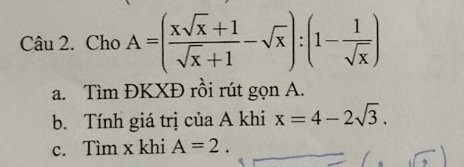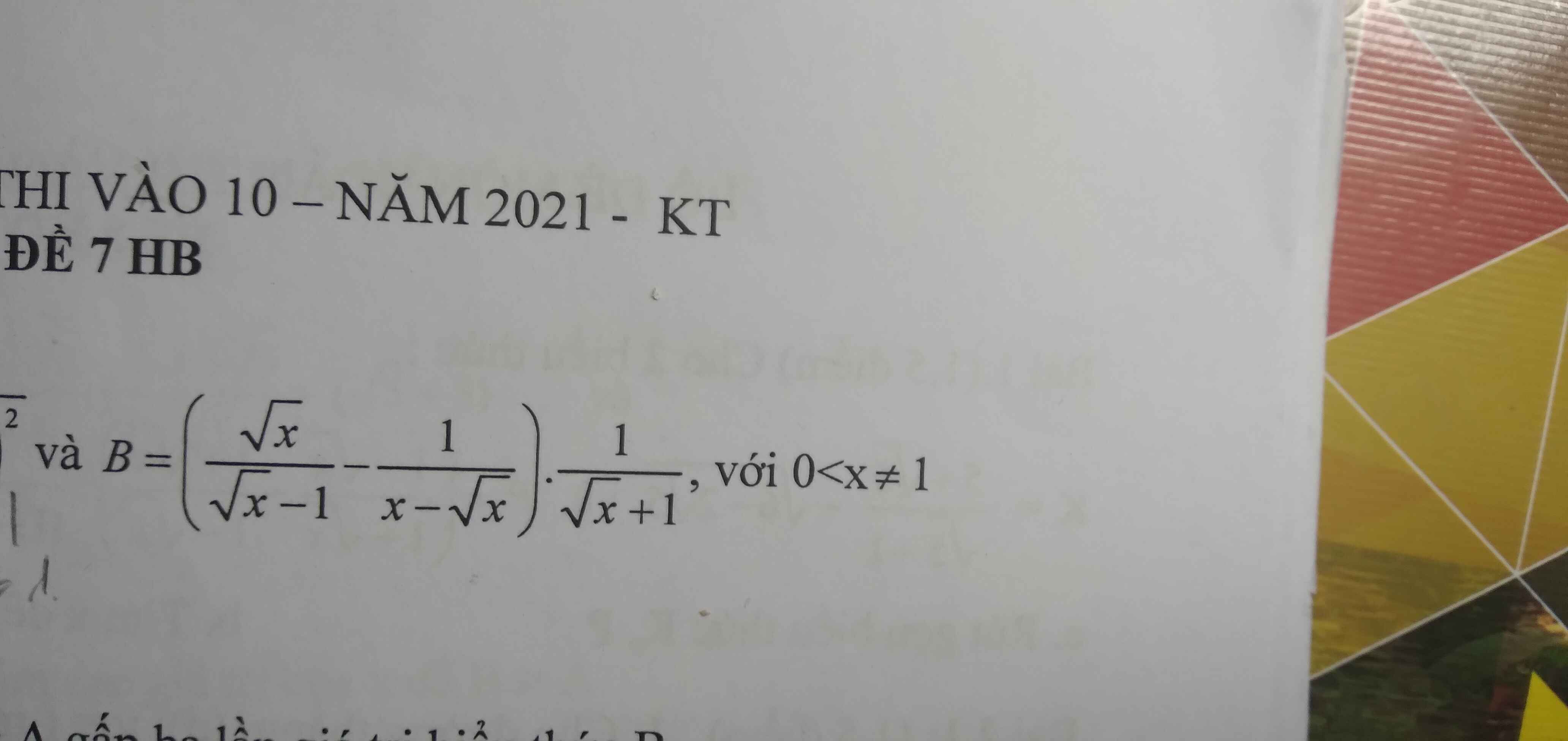Bài 1:
ĐKXĐ: $x>0; x\neq 1$
\(A=\frac{(\sqrt{x}-1)(x+\sqrt{x}+1)}{\sqrt{x}(\sqrt{x}-1)}-\frac{(\sqrt{x}+1)(x-\sqrt{x}+1)}{\sqrt{x}(\sqrt{x}+1)}+\frac{x+1}{\sqrt{x}}\)
\(=\frac{x+\sqrt{x}+1}{\sqrt{x}}-\frac{x-\sqrt{x}+1}{\sqrt{x}}+\frac{x+1}{\sqrt{x}}=\frac{x+\sqrt{x}+1-(x-\sqrt{x}+1)+(x+1)}{\sqrt{x}}\)
\(=\frac{x+2\sqrt{x}+1}{\sqrt{x}}=\frac{(\sqrt{x}+1)^2}{\sqrt{x}}\)
Bài 2:
\(\frac{x+2}{x\sqrt{x}+1}+\frac{\sqrt{x}-1}{x-\sqrt{x}+1}-\frac{\sqrt{x}-1}{x-1}\)
\(=\frac{x+2}{(\sqrt{x}+1)(x-\sqrt{x}+1)}+\frac{(\sqrt{x}-1)(\sqrt{x}+1)}{(\sqrt{x}+1)(x-\sqrt{x}+1)}-\frac{\sqrt{x}-1}{(\sqrt{x}-1)(\sqrt{x}+1)}\)
\(=\frac{x+2+x-1}{(\sqrt{x}+1)(x-\sqrt{x}+1)}-\frac{1}{\sqrt{x}+1}=\frac{2x+1}{(\sqrt{x}+1)(x-\sqrt{x}+1)}-\frac{x-\sqrt{x}+1}{(\sqrt{x}+1)(x-\sqrt{x}+1)}\)
\(=\frac{2x+1-(x-\sqrt{x}+1)}{(\sqrt{x}+1)(x-\sqrt{x}+1)}=\frac{x+\sqrt{x}}{(\sqrt{x}+1)(x-\sqrt{x}+1)}\)
\(=\frac{\sqrt{x}(\sqrt{x}+1)}{(\sqrt{x}+1)(x-\sqrt{x}+1)}=\frac{\sqrt{x}}{x-\sqrt{x}+1}\)
Theo BĐT Cô-si:
$x+1\geq 2\sqrt{x}\Rightarrow x-\sqrt{x}+1\geq \sqrt{x}$
$\Rightarrow B\leq \frac{\sqrt{x}}{\sqrt{x}}=1$
Dấu "=" xảy ra khi $x=1$ (không thỏa mãn vì $x\neq 1$)
$\Leftrightarrow B< 1$
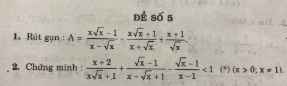 Mn giúp mình bài 2 vs ạ
Mn giúp mình bài 2 vs ạ