a) \(x^2-5x+3=0\)
Ta có: \(\Delta=b^2-4ac=\left(-5\right)^2-4.1.3=13\)
Vì \(\Delta=13>0\Rightarrow\) Phương trình có 2 nghiệm phân biệt
\(x_1=\dfrac{-b+\sqrt{\Delta}}{2a}=\dfrac{-\left(-5\right)+\sqrt{13}}{2.1}=\dfrac{5+\sqrt{13}}{2}\)
\(x_2=\dfrac{-b-\sqrt{\Delta}}{2a}=\dfrac{-\left(-5\right)-\sqrt{13}}{2.1}=\dfrac{5-\sqrt{13}}{2}\)
Vậy phương trình có 2 nghiệm phân biệt \(x_1=\dfrac{5+\sqrt{13}}{2};x_2=\dfrac{5-\sqrt{13}}{2}\)
b) \(-2x^2+4x-1=0\)
Ta có: \(\Delta=b^2-4ac=4^2-4.\left(-2\right).\left(-1\right)=8\)
Vì \(\Delta=8>0\Rightarrow\) Phương trình có 2 nghiệm phân biệt
\(x_1=\dfrac{-b+\sqrt{\Delta}}{2a}=\dfrac{-4+\sqrt{8}}{2.\left(-2\right)}=\dfrac{2-\sqrt{2}}{2}\)
\(x_2=\dfrac{-b-\sqrt{\Delta}}{2a}=\dfrac{-4-\sqrt{8}}{2.\left(-2\right)}=\dfrac{2+\sqrt{2}}{2}\)
Vậy phương trình có 2 nghiệm phân biệt \(x_1=\dfrac{2-\sqrt{2}}{2};x_2=\dfrac{2+\sqrt{2}}{2}\)
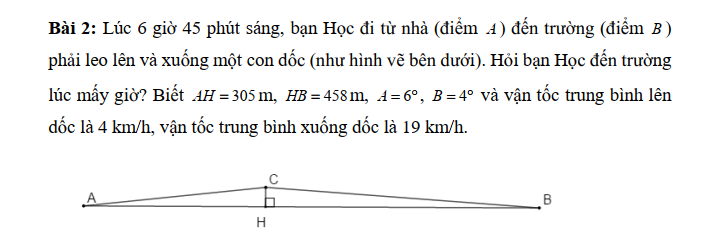
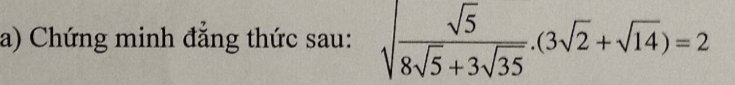
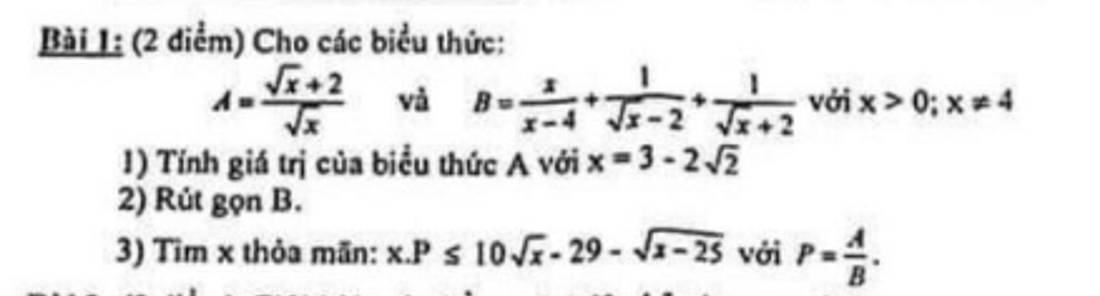
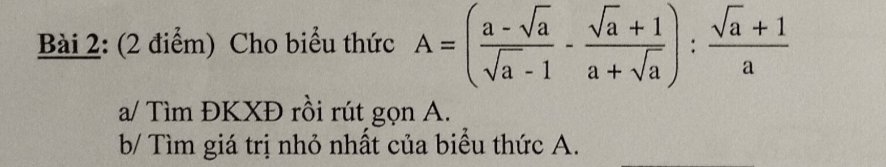
Cho 3 số dương a,b,c thỏa mãn a+b+c=1. CMR: