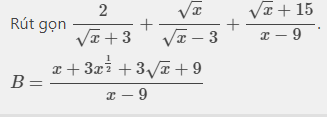\(a,A=\dfrac{4\cdot5}{5+3}=\dfrac{20}{8}=\dfrac{5}{2}\\ b,B=\dfrac{2\sqrt{x}-6+x+3\sqrt{x}+\sqrt{x}+15}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}=\dfrac{x+6\sqrt{x}+9}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\\ B=\dfrac{\left(\sqrt{x}+3\right)^2}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}=\dfrac{\sqrt{x}+3}{\sqrt{x}-3}\\ c,P=AB=\dfrac{4\sqrt{x}}{\sqrt{x}-3}< 0\Leftrightarrow\sqrt{x}-3< 0\Leftrightarrow0\le x< 9\)
Đúng 2
Bình luận (4)
Các câu hỏi tương tự
giải nhanh giúp mình với ạ, nếu được thì giải thích giúp mình với nha, mình cảm ơn 
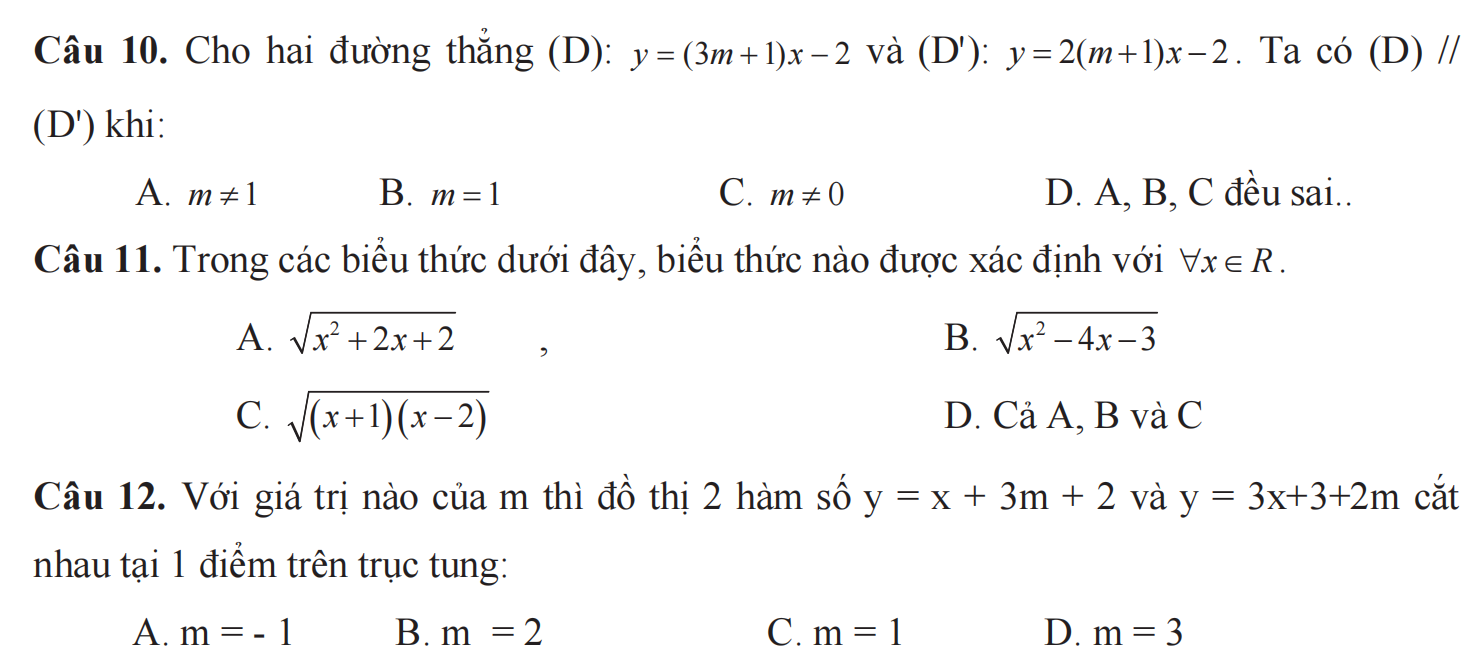

Làm hết giúp mình với các bạn trình bày vẽ hình giúp mình với ạ gấp lắm ạ
giúp mình bài 1 và 2 với mình đang cần gấp. giải ra giúp mình nha

Mình muốn hỏi câu 3 với 4 ai giúp mình với
Giúp mình với mình ạ

Có ai ko giúp mình với. Mình đang cần gấp trước 8h00 . Mong mọi người giúp đỡ. Mình xin cảm ơn ạ
giúp mình với ạ (nếu được giải chi tiết câu a cho mình với mình cảm ơn)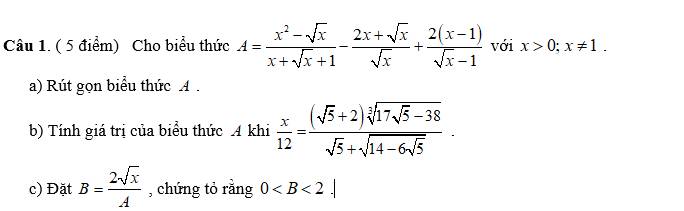

Giúp mình với, mình cần gấpppp
GIÚP MÌNH VỚI MÌNH ĐỘI ƠNN
 giúp mình với mình đang gấp
giúp mình với mình đang gấp