Ta có:
\(C+D=\left(3x^2+2x-8\right)+\left(x^2-2x-8\right)\)
\(=3x^2+2x-8+x^2-2x-8\)
\(=\left(3x^2+x^2\right)+\left(2x-2x\right)-\left(8+8\right)\)
\(=4x^2-16\)
\(=4\left(x^2-4\right)\)
Do đó để đa thức \(C+D\) có nghiệm thì \(4\left(x^2-4\right)=0\)
\(\Rightarrow x^2-4=0\)
\(\Rightarrow x^2=4\)
\(\Rightarrow\left[{}\begin{matrix}x=2\\x=-2\end{matrix}\right.\)
Lại có:
\(C-D=\left(3x^2+2x-8\right)-\left(x^2-2x-8\right)\)
\(=3x^2+2x-8-x^2+2x+8\)
\(=\left(3x^2-x^2\right)+\left(2x+2x\right)-\left(8-8\right)\)
\(=2x^2+4x\)
Do đó để đa thức \(C-D\) có nghiệm thì \(2x^2+4x=0\)
\(\Rightarrow2x\left(x+2\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}2x=0\\x+2=0\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=0\\x=-2\end{matrix}\right.\)
Đúng 1
Bình luận (1)
Các câu hỏi tương tự
Giúp mình với
Ai giúp mình thì cho mình cảm ơn
Giúp mình với các bạn giúp mình rồi mình sẽ giúp các bạn cảm ơn🍜
giúp mình với mình cần gấp
làm nhanh giúp mình nha , mình theo dõi và tick đúng

giúp mình bài này với mình đang cần gấp ( vẽ hình giúp mình luôn nha)
Mâý bạn giúp mình câu 1c với bài 2 giúp mình nha. Mình sắp nộp bài rồi ;-; mình cảm ơn nhiều!
ai giúp mình bài 3 với ạ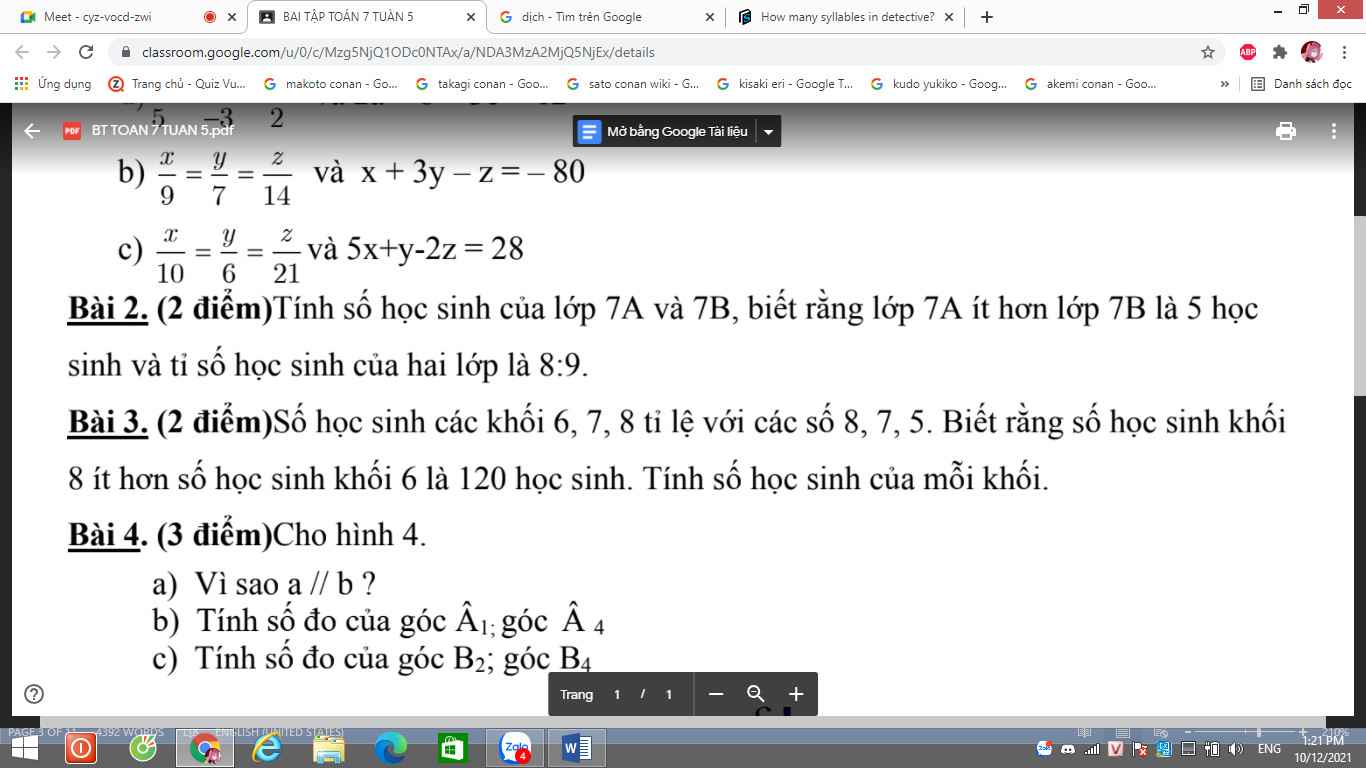 giúp mình với ạ,mình xin cảm ơn trước
giúp mình với ạ,mình xin cảm ơn trước
giúp mình với mn ơi mình cần giúp khẩn cấp T_T
Đọc tiếp
giúp mình với mn ơi mình cần giúp khẩn cấp T_T
Làm giúp mình với
Cảm ơn bạn nào giúp mình nhé

Giúp mình với ạ (bài hình vẽ hình giúp mình ạ)


