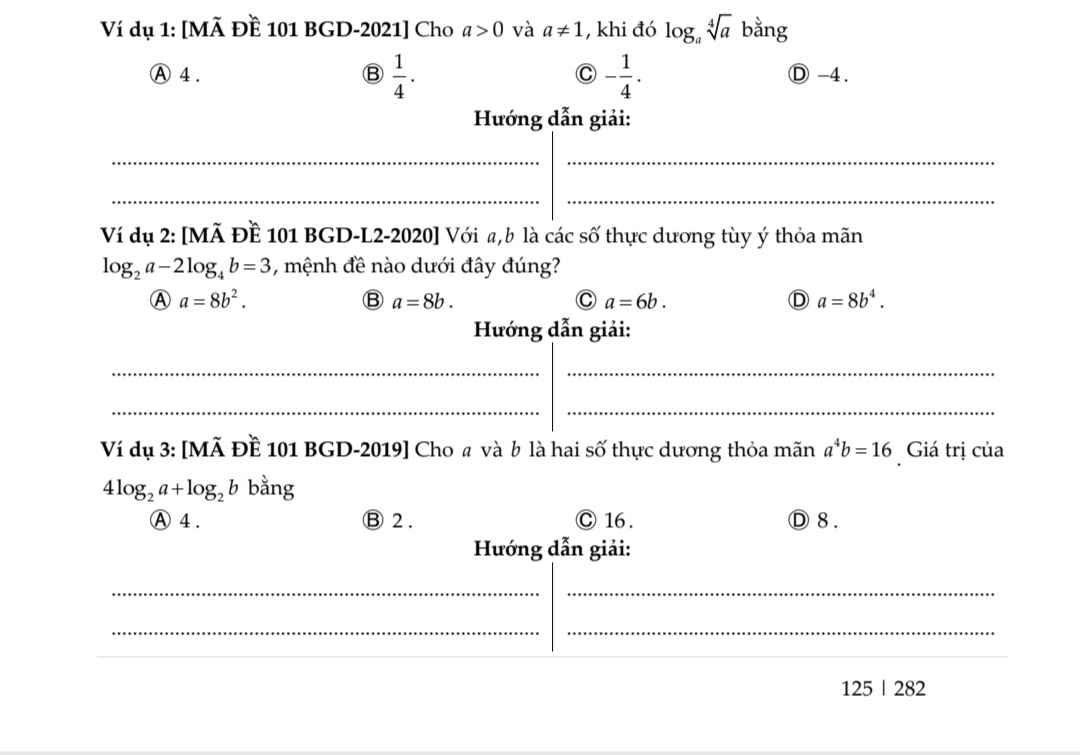
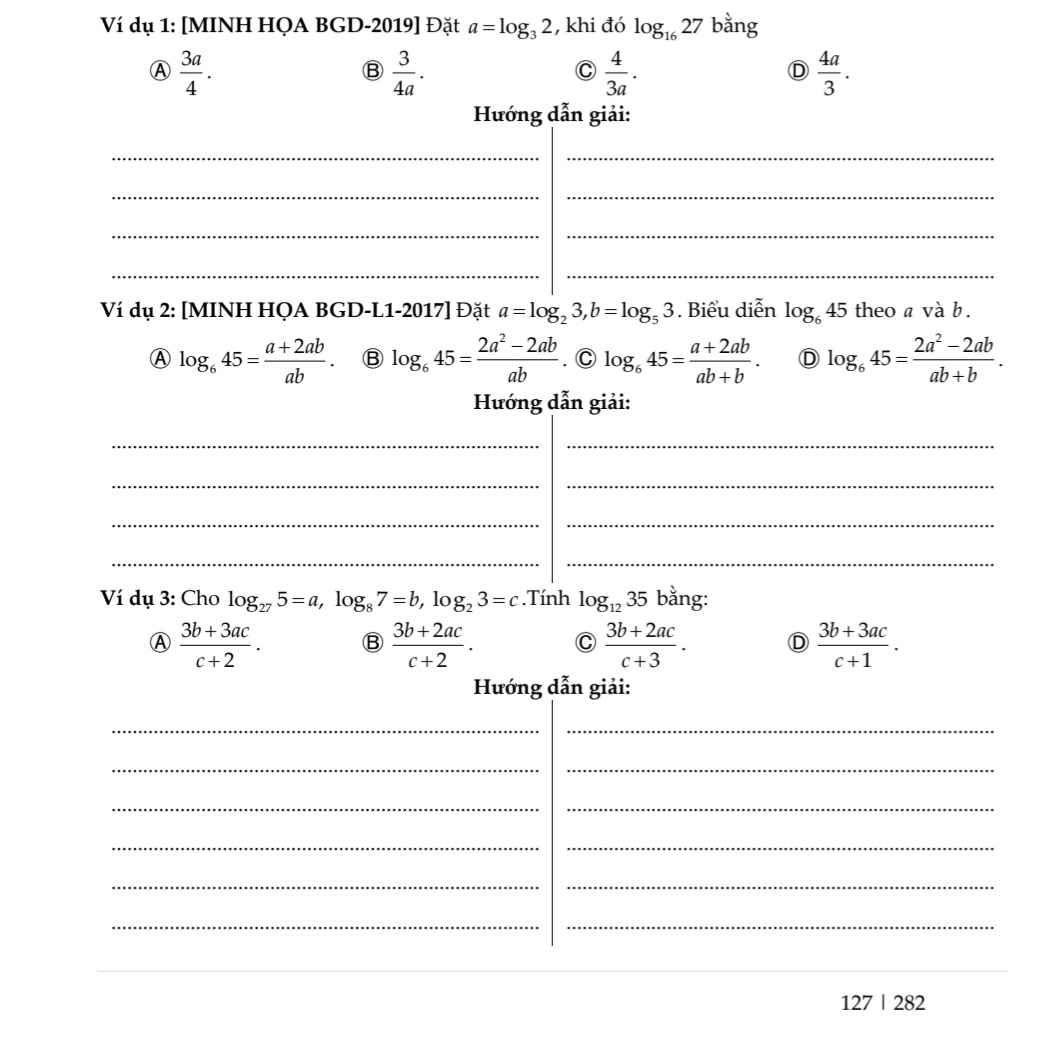
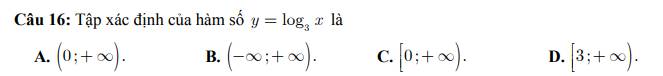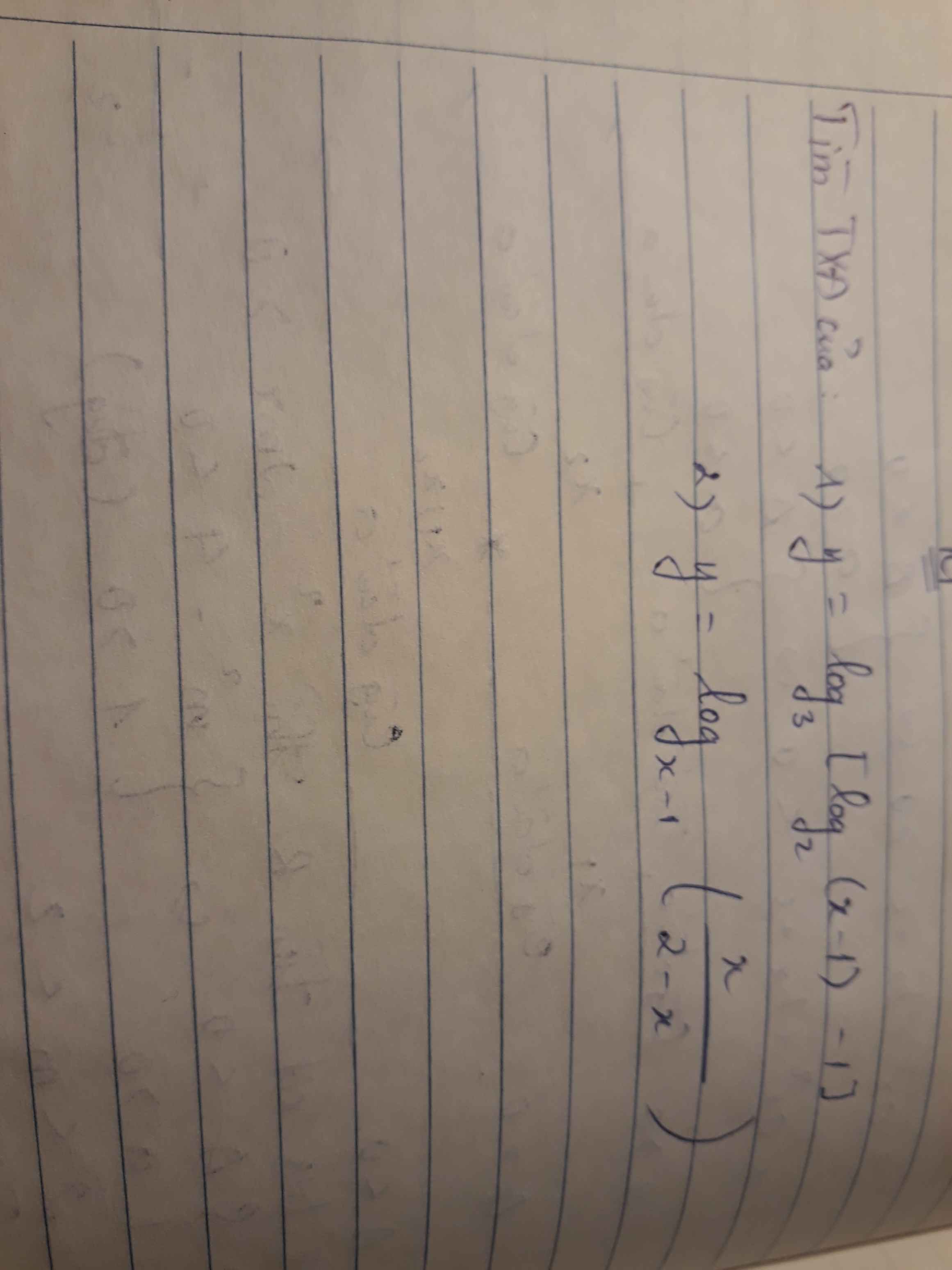Lời giải:
\(f(x)=e^x(\sin x-2\cos x)\)
\(\Rightarrow f'(x)=-e^x\cos x+3e^x\sin x\)
\(f''(x)=4e^x\sin x+2e^x\cos x\)
Do đó:
\(m=\frac{f'(x)}{f''(x)+5e^x}=\frac{-e^x\cos x+3e^x\sin x}{4e^x\sin x+2e^x\cos x+5e^x}=\frac{3\sin x-\cos x}{4\sin x+2\cos +5}\)
\(\Leftrightarrow m(4\sin x+2\cos x+5)=3\sin x-\cos x\)
\(\Leftrightarrow 5m=\sin x(3-4m)+\cos x(-2m-1)\) (*)
Để pt có nghiệm thì \(5m\in [\min; \max]\) của
\(\sin x(3-4m)+\cos x(-2m-1)\) (1)
Áp dụng BĐT Bunhiacopxky:
\([\sin x(3-4m)+\cos x(-2m-1)]^2\leq (\sin^2x+\cos^2x)[(3-4m)^2+(-2m-1)^2](**)\)
\(\Leftrightarrow [\sin x(3-4m)+\cos x(-2m-1)]^2\leq 20m^2-20m+10\)
\(\Leftrightarrow -\sqrt{20m^2-20m+10}\leq \sin x(3-4m)+\cos x(-2m-1)\le \sqrt{20m^2-20m+10}\) (2)
Từ \((1);(2)\Rightarrow -\sqrt{20m^2-20m+10}\leq 5m\leq \sqrt{20m^2-20m+10}\)
\(\Leftrightarrow 25m^2\leq 20m^2-20m+10\) (***)
\(\Leftrightarrow m^2+4m-2\leq 0\Leftrightarrow -2-\sqrt{6}\leq m\leq \sqrt{6}-2\)
Do đó, \(a=-2-\sqrt{6};b=\sqrt{6}-2\)
\(\Leftrightarrow a+4b=-10+3\sqrt{6}\)
Đáp án B
Thực chất bạn có thể kết hợp từ dòng (*), (**), (***) luôn được nhưng để dễ hiểu hơn thì mình biến bài làm dài hơn 1 chút.