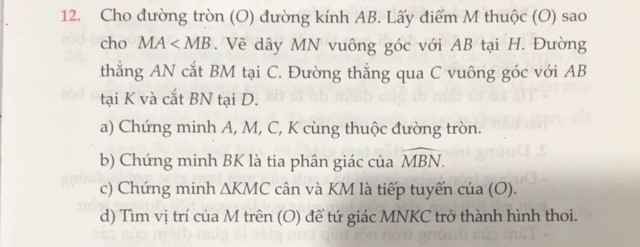
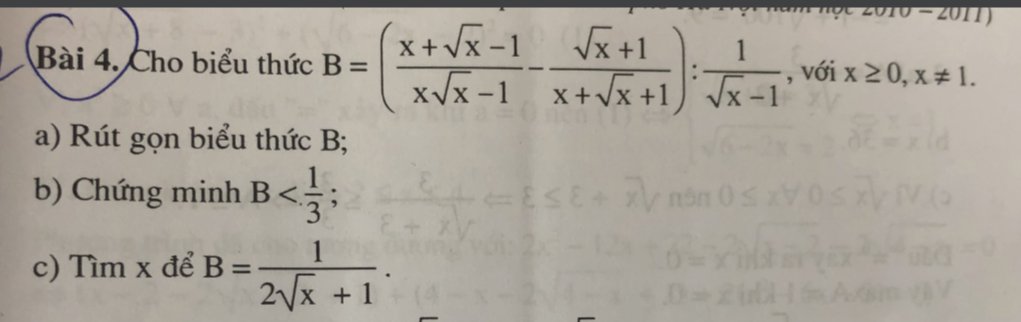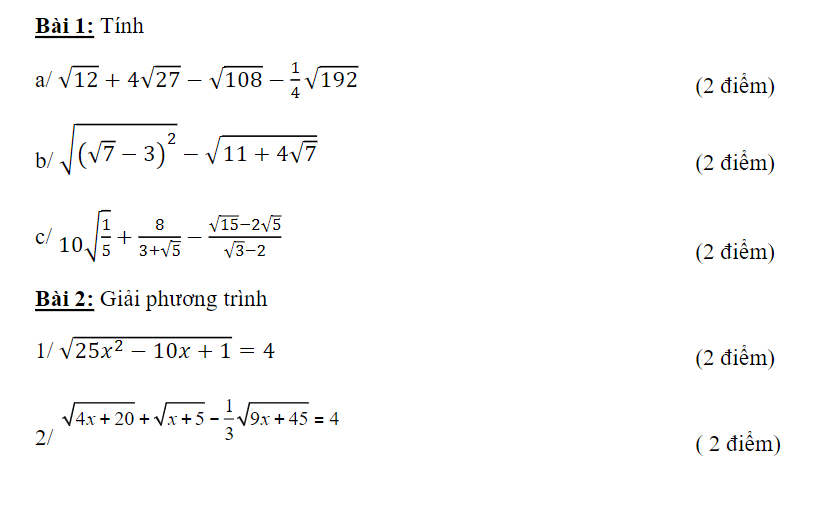\(a,A=\dfrac{\sqrt{4+2\sqrt{3}}}{\sqrt{3}+1}+\dfrac{5+3\sqrt{5}}{\sqrt{5}}-\left(\sqrt{5}+3\right)\\ =\dfrac{\sqrt{\left(\sqrt{3}+1\right)^2}}{\sqrt{3}+1}+\dfrac{\sqrt{5}\left(\sqrt{5}+3\right)}{\sqrt{5}}-\left(\sqrt{5}+3\right)\\ =\dfrac{\left|\sqrt{3}+1\right|}{\sqrt{3}+1}+\left(\sqrt{5}+3\right)-\left(\sqrt{5}+3\right)\\ =\dfrac{\sqrt{3}+1}{\sqrt{3}+1}\\ =1\)
\(b,B=\dfrac{1}{3-\sqrt{x}}+\dfrac{\sqrt{x}}{3+\sqrt{x}}-\dfrac{x+9}{x-9}\left(dk:x\ge0,x\ne9\right)\\ =\dfrac{1}{3-\sqrt{x}}+\dfrac{\sqrt{x}}{3+\sqrt{x}}+\dfrac{x+9}{\left(3-\sqrt{x}\right)\left(3+\sqrt{x}\right)}\\ =\dfrac{3+\sqrt{x}+\sqrt{x}\left(3-\sqrt{x}\right)+x+9}{9-x}\\ =\dfrac{\sqrt{x}+x+12+3\sqrt{x}-x}{9-x}\\ =\dfrac{4\sqrt{x}+12}{9-x}\\ =\dfrac{4\left(\sqrt{x}+3\right)}{\left(3+\sqrt{x}\right)\left(3-\sqrt{x}\right)}\\ =\dfrac{4}{3-\sqrt{x}}\)
\(B>A\Leftrightarrow\dfrac{4}{3-\sqrt{x}}>1\\ \Rightarrow4-3+\sqrt{x}>0\\ \Rightarrow\sqrt{x}>-1\left(LD\right)\)
Kết hợp với điều kiện \(x\ge0,x\ne9\) thì mọi giá trị x còn lại thỏa mãn đề bài.