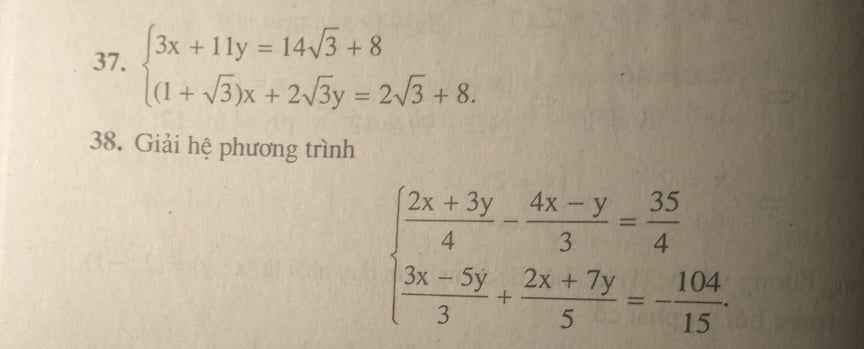b) Ta có: \(\left\{{}\begin{matrix}x-y\sqrt{2}=6\\2\sqrt{2}x-3y=8\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}3x-3\sqrt{2}\cdot y=18\\4x-3\sqrt{2}\cdot y=8\sqrt{2}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-x=18-8\sqrt{2}\\x-y\sqrt{2}=6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=8\sqrt{2}-18\\y\sqrt{2}=x-6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=8\sqrt{2}-18\\y\sqrt{2}=8\sqrt{2}-18-6=8\sqrt{2}-24\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=8\sqrt{2}-18\\y=8-12\sqrt{2}\end{matrix}\right.\)
Vậy: hệ phương trình có nghiệm duy nhất là \(\left\{{}\begin{matrix}x=8\sqrt{2}-18\\y=8-12\sqrt{2}\end{matrix}\right.\)
c) Ta có: \(\left\{{}\begin{matrix}3x+2y=3\\5x+3y=10\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}15x+10y=15\\15x+9y=30\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=-15\\3x+2y=3\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}3x=3-2y=3-2\cdot\left(-15\right)=33\\y=-15\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=11\\y=-15\end{matrix}\right.\)
Vậy: hệ phương trình có nghiệm duy nhất là \(\left\{{}\begin{matrix}x=11\\y=-15\end{matrix}\right.\)
d) Ta có: \(\left\{{}\begin{matrix}x-3y=23\\4x+5y=7\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}4x-12y=92\\4x+5y=7\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-17y=85\\x-3y=23\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}y=-5\\x=23+3y=23+3\cdot\left(-5\right)=8\end{matrix}\right.\)
Vậy: Hệ phương trình có nghiệm duy nhất là (x,y)=(8;-5)