Bài 1:
a. $(3x-1)^{10}=(3x-1)^{20}$
$(3x-1)^{20}-(3x-1)^{10}=0$
$(3x-1)^{10}[(3x-1)^{10}-1]=0$
$\Rightarrow (3x-1)^{10}=0$ hoặc $(3x-1)^{10}=1$
Nếu $(3x-1)^{10}=0$
$\Rightarrow 3x-1=0$
$\Rightarrow x=\frac{1}{3}$
Nếu $(3x-1)^{10}-1=0$
$\Rightarrow 3x-1=1$ hoặc $3x-1=-1$
$\Rightarrow x=\frac{2}{3}$ hoặc $x=0$
b
$x(6-x)^{2003}=(6-x)^{2003}$
$x(6-x)^{2003}-(6-x)^{2003}=0$
$(6-x)^{2003}(x-1)=0$
$\Rightarrow (6-x)^{2003}=0$ hoặc $x-1=0$
$\Rightarrow x=6$ hoặc $x=1$
c.
$5^x+5^{x+2}=650$
$5^x(1+5^2)=650$
$5^x.26=650$
$5^x=25=5^2$
$\Rightarrow x=2$
Bài 2:
a. Trùng với câu c bài 1
b.
$3^2.3^n=3^5$
$3^{n+2}=3^5$
$\Rightarrow n+2=5$
$\Rightarrow n=3$
c.
$(2^2:4).2^n=4$
$1.2^n=4=2^2$
$2^n=2^2$
$\Rightarrow n=2$
Bài 3:
a. $\frac{1}{2}.2^n+4.2^n=9.5^n$
$2^{n-1}+2^{n+2}=9.5^n$
$2^{n-1}(1+2^3)=9.5^n$
$2^{n-1}.9=9.5^n$
$2^{n-1}=5^n$
$(\frac{2}{5})^{n-1}=5$
$n-1=\log_\frac{2}{5}(5)$
(Đề này không phù hợp với lớp 7). Bạn xem lại coi.
b.
$2^n(\frac{1}{2}+4)=9.5^n$
$2^n.\frac{9}{2}=9.5^n$
$2^{n-1}=5^n$ (giống với câu b.
c. Giống với 2 câu còn lại.
Bài 4:
a. $2^{n+3}.2^n=144$
$2^{n+3+n}=144$
$2^{2n+3}=144$
$2n+3=\log_2(144)\approx 7$
$n\approx 2$
b.
$(x-2015)^{x+2}-(x-2015)^{x+12}=0$
$(x-2015)^{x+2}[1-(x-2015)^{10}]=0$
$\Rightarrow (x-2015)^{x+2}=0$ hoặc $1-(x-2015)^{10}=0$
Nếu $(x-2015)^{x+2}=0$
$\Rightarrow x-2015=0$
$\Rightarrow x=2015$
Nếu $1-(x-2015)^{10}=0$
$\Rightarrow x-2015=1$ hoặc $x-2015=-1$
$\Rightarrow x=2016$ hoặc $x=2014$
c.
$(2x-1)^8=(2x-1)^6$
$(2x-1)^8-(2x-1)^6=0$
$(2x-1)^6[(2x-1)^2-1]=0$
$\Rightarrow (2x-1)^6=0$ hoặc $(2x-1)^2-1=0$
Nếu $(2x-1)^6=0$
$\Rightarrow 2x-1=0\Rightarrow x=\frac{1}{2}$
Nếu $(2x-1)^2-1=0$
$\Rightarrow 2x-1=1$ hoặc $2x-1=-1$
$\Rightarrow x=1$ hoặc $x=0$
Bài 5:
$2^{x-1}+5.2^{x-2}=\frac{7}{32}$
$2^{x-2}(2+5)=\frac{7}{32}$
$2^{x-2}.7=\frac{7}{32}$
$2^{x-2}=\frac{7}{32}:7=\frac{1}{32}=2^{-5}$
$\Rightarrow x-2=-5$
$\Rightarrow x=-3$
Bài 6:
a. $(x-7)^{x+1}-(x-7)^{x+11}=0$
$(x-7)^{x+1}[1-(x-7)^{10}]=0$
$\Rightarrow (x-7)^{x+1}=0$ hoặc $1-(x-7)^{10}=0$
Nếu $(x-7)^{x+1}=0$
$\Rightarrow x-7=0\Rightarrow x=7$
Nếu $1-(x-7)^{10}=0$
$\Rightarrow x-7=1$ hoặc $x-7=-1$
$\Rightarrow x=8$ hoặc $x=6$
b.
$2^{x+2}.3^{x+1}.5^x=10800=2^4.3^3.5^2$
$\Rightarrow 2^{x+2-4}.3^{x+1-3}.5^{x-2}=1$
$\Rightarrow 2^{x-2}.3^{x-2}.5^{x-2}=1$
$\Rightarrow (2.3.5)^{x-2}=1$
$\Rightarrow 30^{x-2}=1$
$\Rightarrow x-2=0$
$\Rightarrow x=2$
c.
$(2x-15)^5=(2x-15)^3$
$(2x-15)^5-(2x-15)^3=0$
$(2x-15)^3[(2x-15)^2-1]=0$
$\Rightarrow (2x-15)^3=0$ hoặc $(2x-15)^2-1=0$
$\Rightarrow 2x-15=0$ hoặc $2x-15=1$ hoặc $2x-15=-1$
$\Rightarrow x=\frac{15}{2}$ hoặc $x=8$ hoặc $x=7$




 hình ko cần vẽ đâu ạ, em vẽ rồi. Làm giúp em câu 2b thôi
hình ko cần vẽ đâu ạ, em vẽ rồi. Làm giúp em câu 2b thôi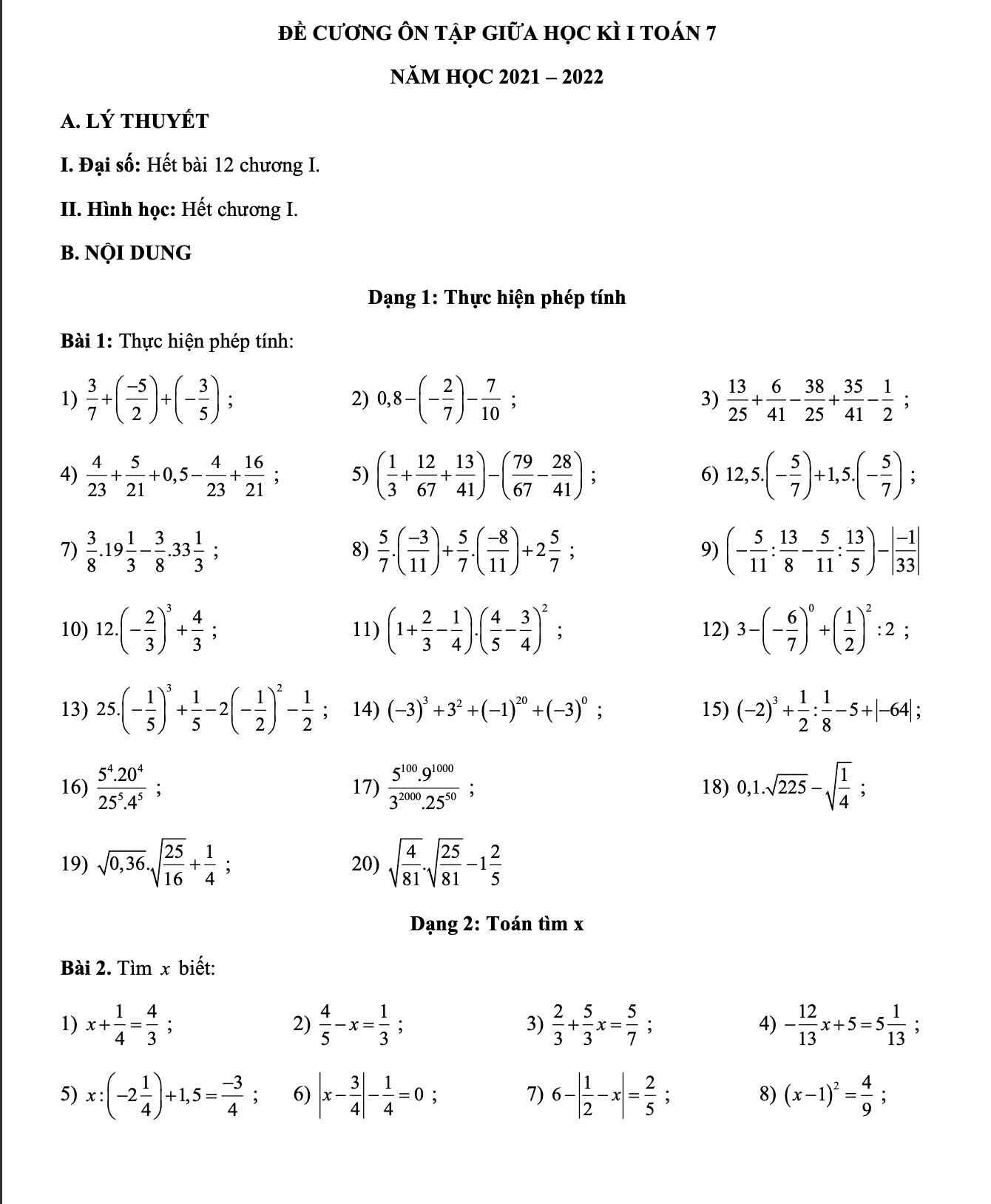
 mn ơi giúp em, nhanh ạ, chỉ cần làm đến bài 2 thôi,bài 1 chỉ cần làm đến câu 17
mn ơi giúp em, nhanh ạ, chỉ cần làm đến bài 2 thôi,bài 1 chỉ cần làm đến câu 17
