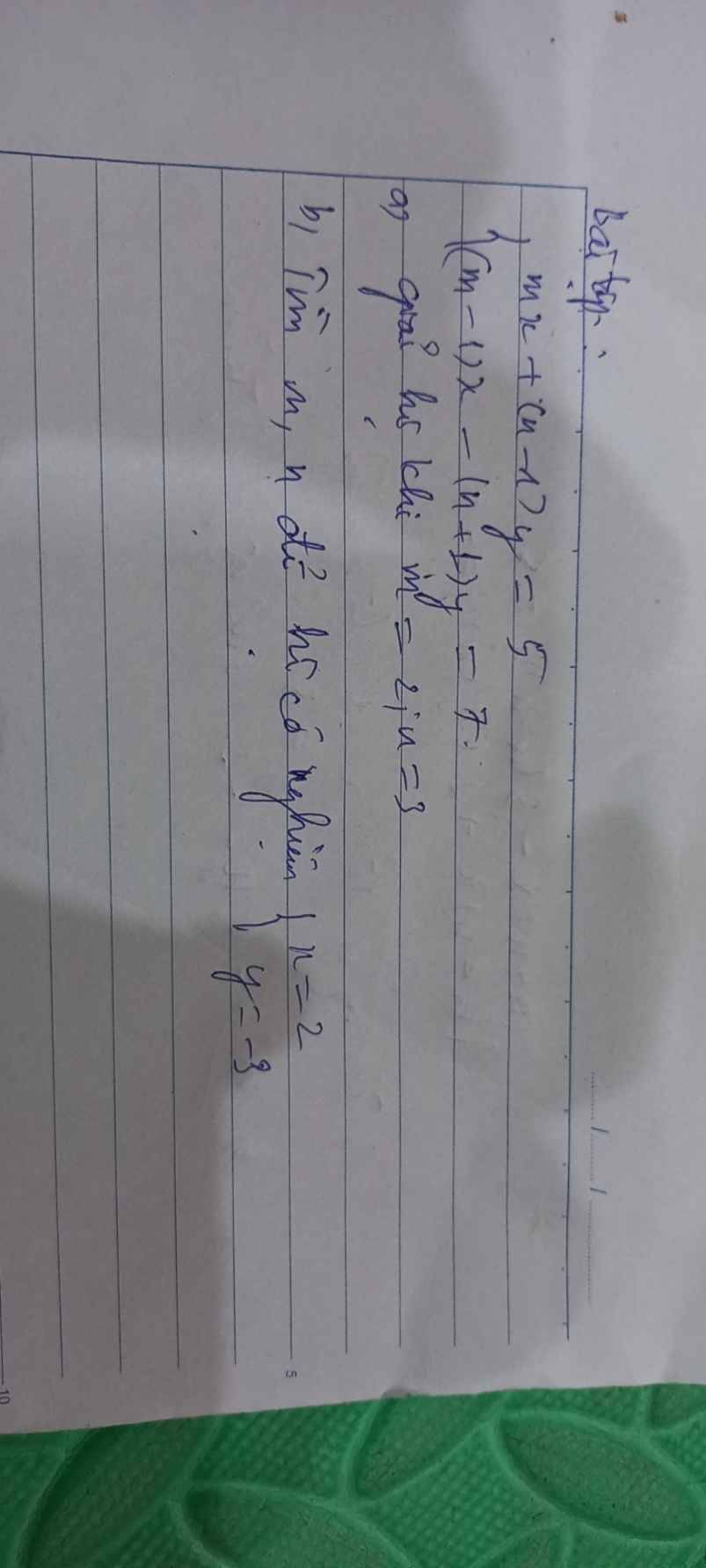a: Thay m=2 và n=3 vào hệ phương trình, ta được:
\(\left\{{}\begin{matrix}2x+\left(3-1\right)y=5\\\left(2-1\right)x-\left(3+1\right)y=7\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2x+2y=5\\x-4y=7\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2x+2y=5\\2x-8y=14\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}10y=-9\\x-4y=7\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=-\dfrac{9}{10}\\x=4y+7=4\cdot\dfrac{-9}{10}+7=\dfrac{-36+70}{10}=\dfrac{34}{10}=\dfrac{17}{5}\end{matrix}\right.\)
b: Thay x=2 và y=-3 vào hệ phương trình, ta được:
\(\left\{{}\begin{matrix}2m+\left(n-1\right)\cdot\left(-3\right)=5\\2\left(m-1\right)-\left(n+1\right)\cdot\left(-3\right)=7\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2m-3n+3=5\\2m-2+3n-3=7\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2m-3n=2\\2m+3n=7+2+3=12\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}4m=14\\2m-3n=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m=\dfrac{7}{2}\\3n=2m+2=2\cdot\dfrac{7}{2}+2=9\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}m=\dfrac{7}{2}\\n=3\end{matrix}\right.\)