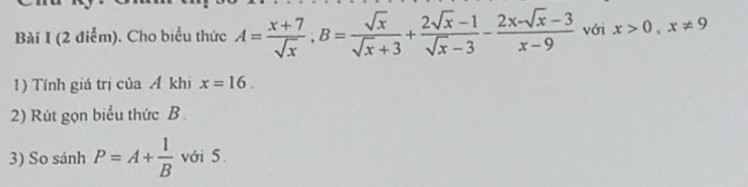\(a,A=\left(\dfrac{\sqrt{x}}{\sqrt{x}-1}+\dfrac{1}{x-\sqrt{x}}\right):\left(\dfrac{1}{\sqrt{x}+1}+\dfrac{2}{x-1}\right)\left(x>0,x\ne1\right)\)
\(=\left(\dfrac{\sqrt{x}}{\sqrt{x}-1}+\dfrac{1}{\sqrt{x}\left(\sqrt{x}-1\right)}\right):\left(\dfrac{1}{\sqrt{x}+1}+\dfrac{2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\right)\)
\(=\dfrac{x+1}{\sqrt{x}\left(\sqrt{x}-1\right)}.\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\sqrt{x}+1}\)
\(=\dfrac{\left(x+1\right)\left(\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}+1\right)}\)
\(=\dfrac{x+1}{\sqrt{x}}\)
Vậy \(A=\dfrac{x+1}{\sqrt{x}}\)
\(b,\) \(A=2\Leftrightarrow\dfrac{x+1}{\sqrt{x}}=2\)
\(\Leftrightarrow\dfrac{x+1-2\sqrt{x}}{\sqrt{x}}=0\)
\(\Leftrightarrow x+1-2\sqrt{x}=0\)
\(\Leftrightarrow x-2\sqrt{x}=-1\)
\(\Leftrightarrow x=1\)
Vậy khi \(A=2\) thì \(x=1\)
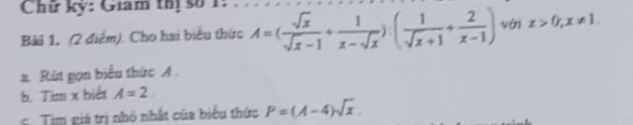


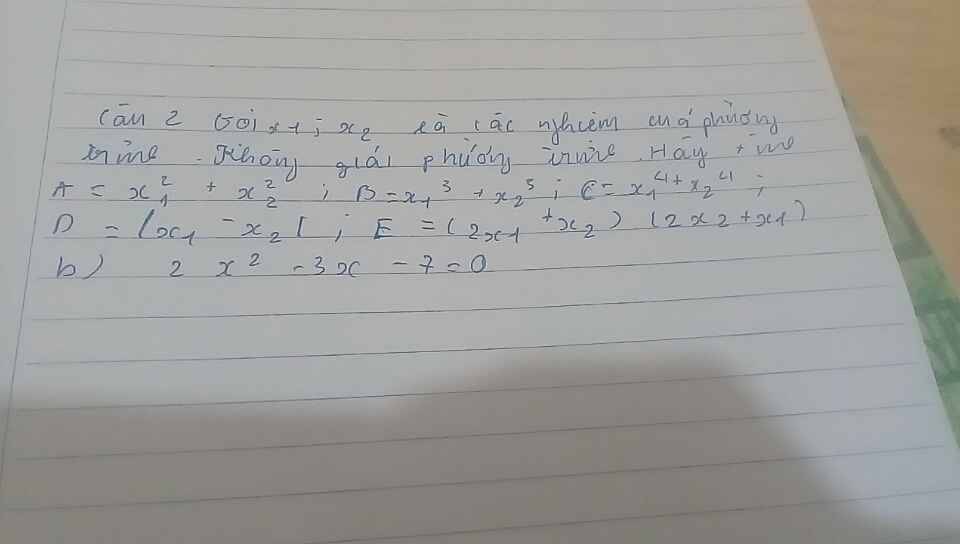

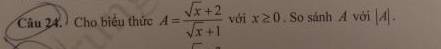

 giup minh cau nay voi
giup minh cau nay voi giup minh cau nay voi
giup minh cau nay voi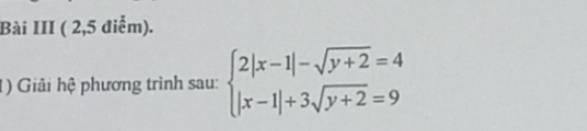
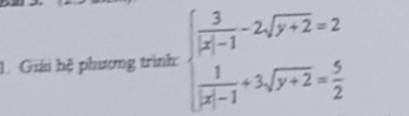 giup minh cau nay voi
giup minh cau nay voi