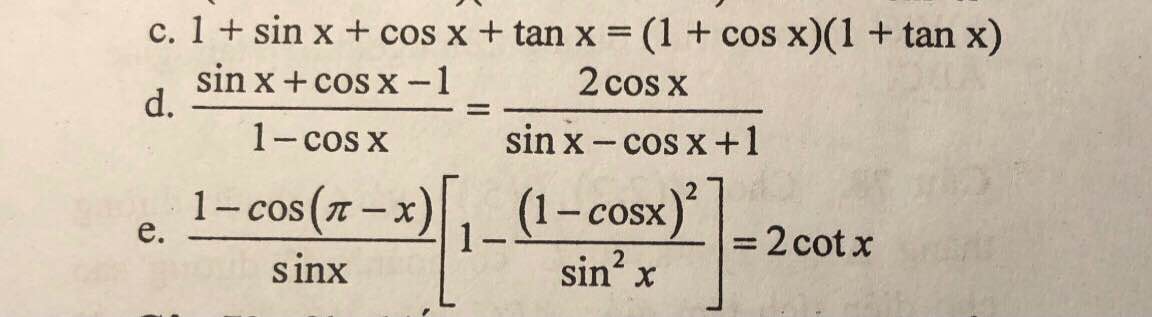c.
\(1+sinx+cosx+tanx=1+sinx+cosx+\dfrac{sinx}{cosx}\)
\(=1+cosx+sinx\left(1+\dfrac{1}{cosx}\right)=1+cosx+\dfrac{sinx\left(1+cosx\right)}{cosx}\)
\(=\left(1+cosx\right)\left(1+\dfrac{sinx}{cosx}\right)=\left(1+cosx\right)\left(1+tanx\right)\)
d.
\(\dfrac{sinx+cosx-1}{1-cosx}=\dfrac{\left(sinx+cosx-1\right)\left(sinx-cosx+1\right)}{\left(1-cosx\right)\left(sinx-cosx+1\right)}\)
\(=\dfrac{sin^2x-\left(cosx-1\right)^2}{\left(1-cosx\right)\left(sinx-cosx+1\right)}=\dfrac{sin^2x-cos^2x+2cosx-1}{\left(1-cosx\right)\left(sinx-cosx+1\right)}\)
\(=\dfrac{-2cos^2x+2cosx}{\left(1-cosx\right)\left(sinx-cosx+1\right)}=\dfrac{2cosx\left(1-cosx\right)}{\left(1-cosx\right)\left(sinx-cosx+1\right)}\)
\(=\dfrac{2cosx}{sinx-cosx+1}\)
e.
\(=\dfrac{1+cosx}{sinx}\left[\dfrac{sin^2x-\left(1-2cosx+cos^2x\right)}{sin^2x}\right]=\dfrac{1+cosx}{sinx}\left[\dfrac{1-cos^2x-1+2cosx-cos^2x}{sin^2x}\right]\)
\(=\dfrac{1+cosx}{sinx}\left[\dfrac{2cosx-2cos^2x}{sin^2x}\right]=\dfrac{2cosx\left(1-cosx\right)\left(1+cosx\right)}{sin^3x}=\dfrac{2cosx.\left(1-cos^2x\right)}{sin^3x}\)
\(=\dfrac{2cosx.sin^2x}{sin^3x}=\dfrac{2cosx}{sinx}=2cotx\)