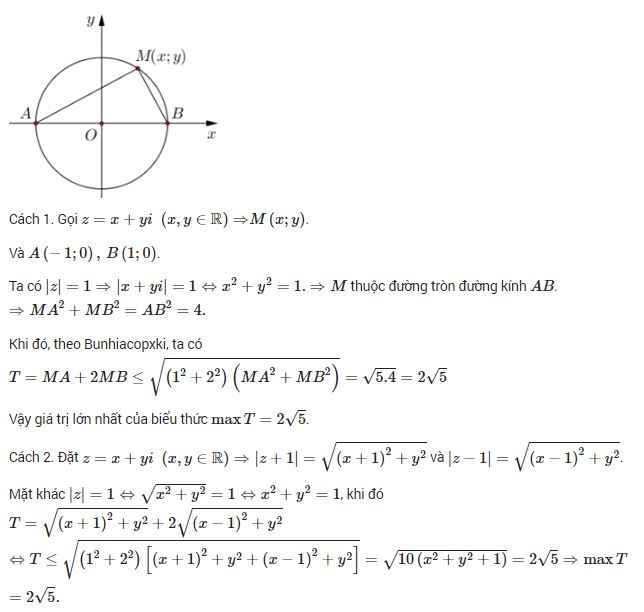
Có thể đưa về hàm số:
\(AB=2\Rightarrow MB=\sqrt{AB^2-MA^2}=\sqrt{4-MA^2}\)
Đặt \(MA=t\) với \(0\le t\le2\) \(\Rightarrow MB=\sqrt{4-t^2}\)
\(P=MA+2MB=f\left(t\right)=t+2\sqrt{4-t^2}\)
Xét hàm \(f\left(t\right)\) trên \(\left[0;2\right]\)
\(f'\left(t\right)=1-\dfrac{2t}{\sqrt{4-t^2}}=0\Rightarrow2t=\sqrt{4-t^2}\Rightarrow5t^2=4\Rightarrow t=\dfrac{2}{\sqrt{5}}\)
\(f\left(0\right)=4\) ; \(f\left(2\right)=2\) ; \(f\left(\dfrac{2}{\sqrt{5}}\right)=2\sqrt{5}\)
\(\Rightarrow f\left(t\right)_{max}=2\sqrt{5}\Rightarrow P_{max}=2\sqrt{5}\)