2:
a: pi/2<a<pi
=>cosa<0
sin^2a+cos^2a=1
=>cos^2a=1-4/9=5/9
=>cosa=-căn 5/3
cos2a=2*cos^2a-1=2*5/9-1=10/9-1=1/9
sin(2a-pi/6)
=sin2a*cospi/6-cos2a*sinpi/6
=2*sina*cosa*(căn 3/2)-1/9*1/2
\(=2\cdot\dfrac{2}{3}\cdot\dfrac{-\sqrt{5}}{3}\cdot\dfrac{\sqrt{3}}{2}-\dfrac{1}{18}=\dfrac{-4\sqrt{15}-1}{18}\)
b; tan a=2
=>sin a=2*cosa
\(A=\dfrac{3\cdot\left(2\cdot cosa\right)^2-cos^2a+2}{5\cdot\left(2\cdot cosa\right)^2+3cosa\cdot2cosa}\)
\(=\dfrac{12\cdot cos^2a-cos^2a+2}{20cos^2a+6cos^2a}\)
\(=\dfrac{11cos^2a+2\left(4cos^2a+cos^2a\right)}{26cos^2a}=\dfrac{21}{26}\)
4:
a: (C): x^2+y^2-4x+2y-4=0
=>x^2-4x+4+y^2+2y+1=9
=>(x-2)^2+(y+1)^2=9
=>I(2;-1); R=3
b: Gọi (d) là phương trình cần tìm
(d)//4x+3y-1=0
=>(d): 4x+3y+c=0
I(2;-1);R=3
Theo đề, ta có: d(I;(d))=R=3
=>\(\dfrac{\left|4\cdot2+3\cdot\left(-1\right)+c\right|}{\sqrt{4^2+3^2}}=3\)
=>|c+5|=15
=>c=10 hoặc c=-20
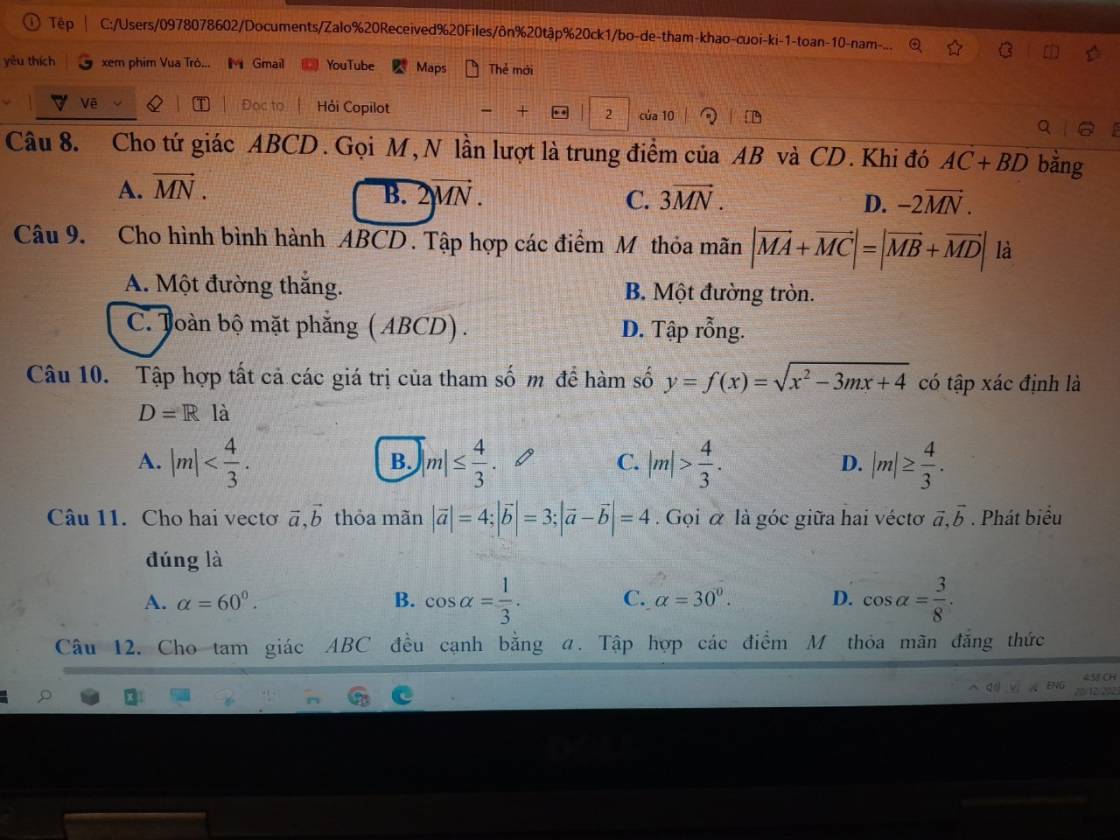giúp tớ câu 9 với ạ