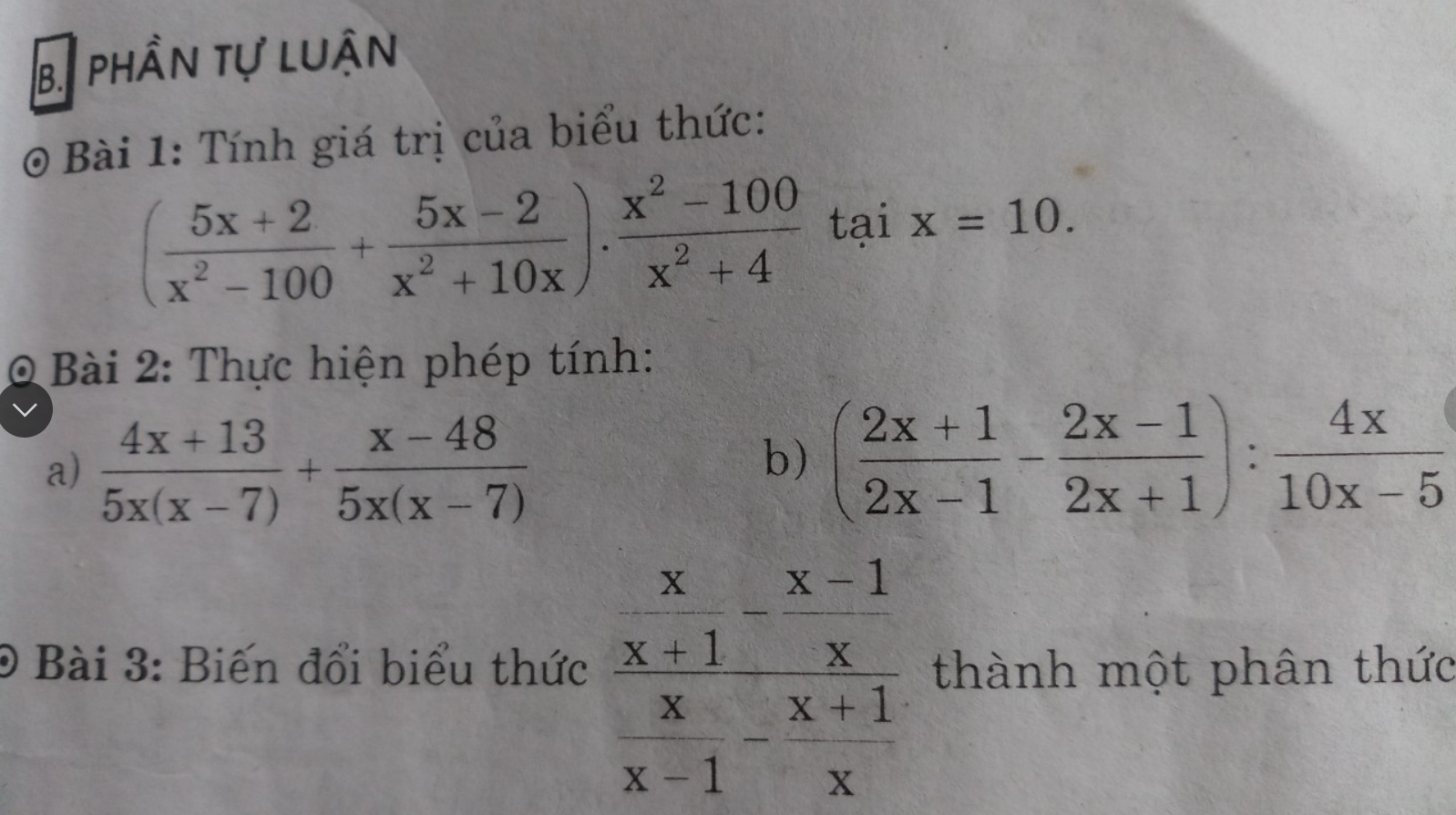Bài 1:
\(=\dfrac{5x^2+2x+5x^2-52x+20}{x\left(x-10\right)\left(x+10\right)}\cdot\dfrac{\left(x-10\right)\left(x+10\right)}{x^2+4}\\ =\dfrac{10x^2-50x+20}{x\left(x^2+4\right)}=\dfrac{10\left(x^2-5x+2\right)}{x\left(x^2+4\right)}\\ =\dfrac{10\left(100-50+2\right)}{10\left(100+4\right)}=\dfrac{52}{104}=\dfrac{1}{2}\)
Bài 2:
\(a,=\dfrac{4x+13+x-48}{5x\left(x-7\right)}=\dfrac{5\left(x-7\right)}{5x\left(x-7\right)}=\dfrac{1}{x}\\ b,=\dfrac{4x^2+4x+1-4x^2+4x-1}{\left(2x-1\right)\left(2x+1\right)}\cdot\dfrac{5\left(2x-1\right)}{4x}\\ =\dfrac{40x}{4x\left(2x+1\right)}=\dfrac{10}{2x+1}\)
Bài 3:
\(=\dfrac{x^2-x^2+1}{x\left(x+1\right)}:\dfrac{x^2-x^2+1}{x\left(x-1\right)}=\dfrac{1}{x\left(x+1\right)}\cdot x\left(x-1\right)=\dfrac{x-1}{x+1}\)