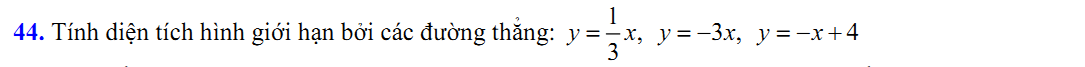Lời giải:
a. TXĐ: $D=\mathbb{R}$
b.
ĐKXĐ: $x^2-6x+10\neq 0$
$\Leftrightarrow (x-3)^2+1\neq 0$
$\Leftrightarrow (x-3)^2\neq -1$ (luôn đúng với mọi $x\in\mathbb{R}$
Vậy TXĐ: $D=\mathbb{R}$
c.
\(\left\{\begin{matrix} x-1\geq 0\\ |x|-2\neq 0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq 1\\ x\neq \pm 2\end{matrix}\right.\Leftrightarrow x\geq 1; x\neq 2\)
d.
\(\left\{\begin{matrix} x\geq 0\\ 2-x\geq 0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq 0\\ x\leq 2\end{matrix}\right.\Leftrightarrow 0\leq x\leq 2\)
e.
\(\left\{\begin{matrix} x^2-9\geq 0\\ x-2\neq 0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} \left[\begin{matrix} x\geq 3\\ x\leq -3\end{matrix}\right.\\ x\neq 2\end{matrix}\right.\)
\(\Leftrightarrow \left[\begin{matrix} x\geq 3; x\neq 2\\ x\leq -3\end{matrix}\right.\)