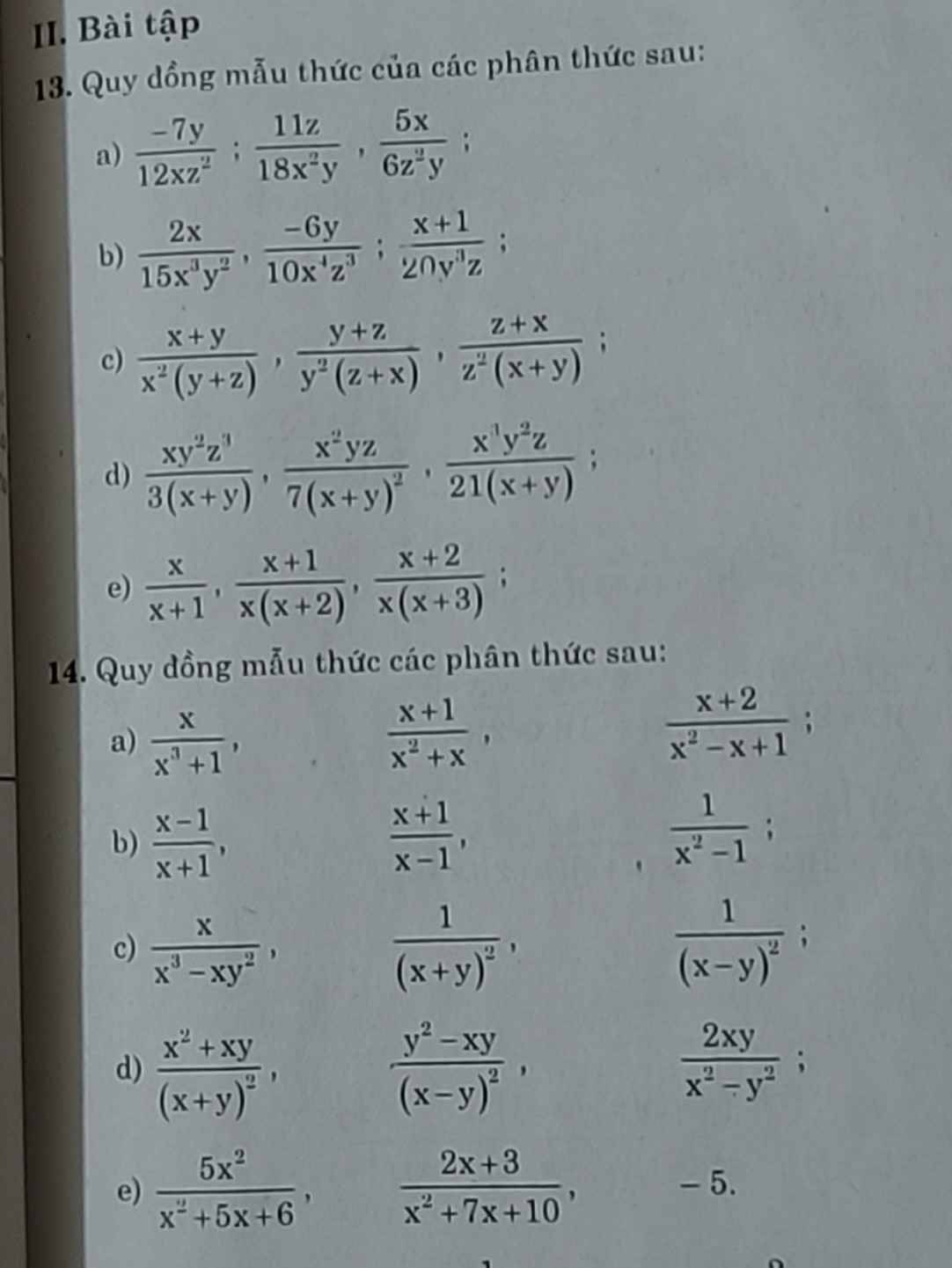14:
a: \(\dfrac{x}{x^3+1}=\dfrac{x}{\left(x+1\right)\left(x^2-x+1\right)}=\dfrac{x^2}{x\left(x+1\right)\left(x^2-x+1\right)}\)
\(\dfrac{x+1}{x^2+x}=\dfrac{1}{x}=\dfrac{x^3+1}{x\left(x+1\right)\left(x^2-x+1\right)}\)
\(\dfrac{x+2}{x^2-x+1}=\dfrac{x\left(x+2\right)\left(x+1\right)}{x\left(x+1\right)\left(x^2-x+1\right)}\)
b: \(\dfrac{x-1}{x+1}=\dfrac{\left(x-1\right)^2}{\left(x+1\right)\left(x-1\right)}\)
\(\dfrac{x+1}{x-1}=\dfrac{\left(x+1\right)^2}{\left(x-1\right)\left(x+1\right)}\)
\(\dfrac{1}{x^2-1}=\dfrac{1}{\left(x+1\right)\left(x-1\right)}\)
c: \(\dfrac{x}{x^3-xy^2}=\dfrac{x}{x\left(x-y\right)\left(x+y\right)}=\dfrac{1}{\left(x-y\right)\left(x+y\right)}=\dfrac{x^2-y^2}{\left(x-y\right)^2\cdot\left(x+y\right)^2}\)
\(\dfrac{1}{\left(x+y\right)^2}=\dfrac{\left(x-y\right)^2}{\left(x-y\right)^2\cdot\left(x+y\right)^2}\)
\(\dfrac{1}{\left(x-y\right)^2}=\dfrac{\left(x+y\right)^2}{\left(x-y\right)^2\cdot\left(x+y\right)^2}\)
d: \(\dfrac{x^2+xy}{\left(x+y\right)^2}=\dfrac{x\left(x+y\right)}{\left(x+y\right)^2}=\dfrac{x}{x+y}=\dfrac{x^2-xy}{\left(x+y\right)\left(x-y\right)}\)
\(\dfrac{y^2-xy}{\left(x-y\right)^2}=\dfrac{-y\left(x-y\right)}{\left(x-y\right)^2}=\dfrac{-y}{x-y}=\dfrac{-y\left(x+y\right)}{\left(x-y\right)\left(x+y\right)}\)
\(\dfrac{2xy}{x^2-y^2}=\dfrac{2xy}{\left(x-y\right)\left(x+y\right)}\)
e: \(\dfrac{5x^2}{x^2+5x+6}=\dfrac{5x^2\left(x+5\right)}{\left(x+5\right)\left(x+2\right)\left(x+3\right)}\)
\(\dfrac{2x+3}{x^2+7x+10}=\dfrac{\left(2x+3\right)\left(x+3\right)}{\left(x+2\right)\left(x+5\right)\left(x+3\right)}\)
\(-5=\dfrac{-5\left(x+2\right)\left(x+5\right)\left(x+3\right)}{\left(x+2\right)\left(x+5\right)\left(x+3\right)}\)