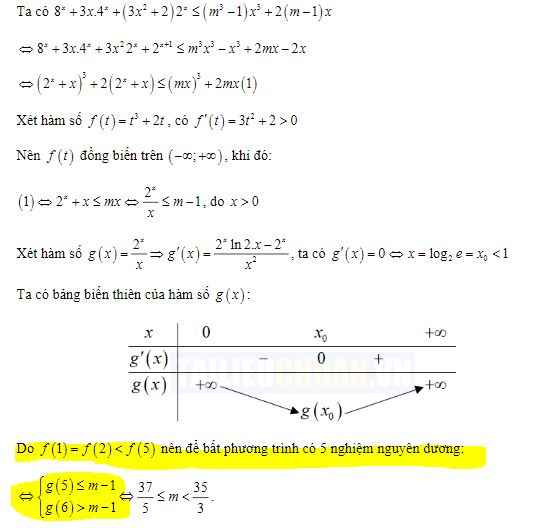
\(\left(x^3-3x+2\right)^2=m\left(x^3-3x+2\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}x^3-3x+2=0\\x^3-3x+2=m\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=-2\\x=1\\x^3-3x+2=m\end{matrix}\right.\)
Xét hàm \(f\left(x\right)=x^3-3x+2\Rightarrow f'\left(x\right)=3x^2-3=0\Rightarrow x=\pm1\)
\(f\left(-1\right)=4\) ; \(f\left(1\right)=0\)
Do đề bài ko yêu cầu 5 nghiệm thực phân biệt nên chỉ cần đường thẳng \(y=m\) cắt đồ thị \(y=f\left(x\right)\) tại 3 điểm
\(\Rightarrow0\le m\le4\) (tại 0 và 4 thì \(y=m\) và \(y=f\left(x\right)\) có 1 nghiệm đơn và 1 nghiệm kép, tính là 3 nghiệm)