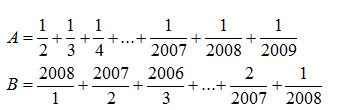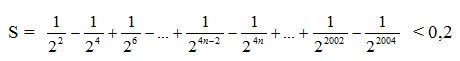Bài toán 2. Tính tỉ số , biết:Bài toán 3. Tìm x; y biết:a. . 25 – y2 8( x – 2009)b. x3 y x y3 + 1997c. x + y + 9 xy – 7.Bài toán 4. Cho n số x1, x2, ..., xn mỗi số nhận giá trị 1 hoặc -1. Chứng minh rằng nếu x1.x2 + x2.x3 + ...+ xn.x1 0 thì n chia hết cho 4.Bài toán 5. Chứng minh rằng:Bài toán 6. Tìm tổng các hệ số của đa thức nhận được sau khi bỏ dấu ngoặc trong biểu thức: A(x) ( 3 - 4x + x2 )2004 .( 3 + 4x + x2 )2005Bài toán 7. Cho a là số gồm 2n chữ số 1, b là số gồm n + 1 chữ số 1, c l...
Đọc tiếp
Bài toán 2. Tính tỉ số  , biết:
, biết:
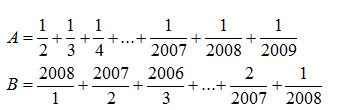
Bài toán 3. Tìm x; y biết:
a. . 25 – y2 = 8( x – 2009)
b. x3 y = x y3 + 1997
c. x + y + 9 = xy – 7.
Bài toán 4. Cho n số x1, x2, ..., xn mỗi số nhận giá trị 1 hoặc -1. Chứng minh rằng nếu x1.x2 + x2.x3 + ...+ xn.x1 = 0 thì n chia hết cho 4.
Bài toán 5. Chứng minh rằng:
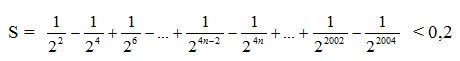
Bài toán 6. Tìm tổng các hệ số của đa thức nhận được sau khi bỏ dấu ngoặc trong biểu thức: A(x) = ( 3 - 4x + x2 )2004 .( 3 + 4x + x2 )2005
Bài toán 7. Cho a là số gồm 2n chữ số 1, b là số gồm n + 1 chữ số 1, c là số gồm n chữ số 6. Chứng minh rằng a + b + c + 8 là số chính phương.
Bài toán 8. Chứng minh rằng với mọi số tự nhiên a, tồn tại số tự nhiên b sao cho ab + 4 là số chính phương.
Bài toán 9. Cho hai số tự nhiên a và b (a < b). Tìm tổng các phân số tối giản có mẫu bằng 7, mỗi phân số lớn hơn a nhưng nhỏ hơn b.
Bài toán 10. Chứng minh rằng: A = 1 + 3 + 5 + 7 + ... + n là số chính phương (n lẻ).
Bài toán 11. Tìm n biết rằng: n3 - n2 + 2n + 7 chia hết cho n2 + 1.
Bài toán 12. Tìm số tự nhiên n để 1n + 2n + 3n + 4n chia hết cho 5.
làm ơn giúp mình